2016 Penny Challenge
@mawhite (242)
United States
January 1, 2016 6:56am CST
I have seen various numbers as the end result for the Penny Challenge that has been going around Facebook.
In a nutshell, the challenge works like this: You start off today, by putting $0.01 into a jar. Every day, you add $0.01 to the amount you save. So, tomorrow would be $0.02, the next day would be $0.03, and so on. By the end of the year, if you do this regularly, you'll have over $667.
It's what's comes after the $667 though that seems to have my math thrown off. The post I saw said at the end of the year, the amount would be $667.90. I did a spreadsheet that came up with an end result of $667.05. I've also seen people come up other numbers as well.
Math has never been my strong point!
In any event, I'm going to give it a try, and say whatever the end amount is, on January 2, 2017, I'll open a CD with whatever it is!
If you want to check (and hopefully correct!!) my math, my spreadsheet is at the link below:
JavaScript isn't enabled in your browser, so this file can't be opened. Enable and reload.Sign inThe Penny Challenge ShareThis version of Firefox is no longer supported. Please upgrade to a supported browser.DismissFileEditViewInsertFormatDataToolsFormAd
8 people like this
8 responses
@owlwings (43910)
• Cambridge, England
1 Jan 16
There are 366 days in 2016 (because it's a leap year), so on December 31st 2016, you will be due to put 366 pennies (i.e. $3.66) in your pot.
If you lined up the pennies you save each day, you would have 1 in the first row, 2 in the second and so on until you get to 366 in the last row.
You can easily see that this arrangement is what is called a triangle number and there is a simple formula for the sums of triangle numbers which says that the series:
1+2+3+4+5...+n = n*(n+1)/2
as n in this case is equal to 366, (366*367)/2 is the number of pennies you should have in your pot at the end of the year. That works out to $671.61.
If there were 365 days in the year (as there are in any year but a leap year) the amount you would save would be $667.95 (try the math for yourself).
I had some trouble loading your spreadsheet (I'm not sure what the problem was: it loaded but then gave me an error), so I wasn't able to check it in detail to see what went wrong with your calculation. Did you forget February 29th? It appears that a lot of people have made similar errors, anyway!
Here's the explanation (if you want it) for the triangle number formula:
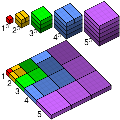
From Wikipedia, the free encyclopedia Jump to: navigation, search The first six triangular numbers A triangular number or triangle number counts the objects that can form an equilateral triangle, as in the diagram on the right. The nth triangular number is
3 people like this
@mawhite (242)
• United States
1 Jan 16
OK now, number one because you were SO THOUGHTFUL to take the time to really explain this in great detail, and number two, because I am determined to understand this, here is the one point where I'm messing up.
I read over the Wikipedia article, and I read your comment a few times, but I'm still feeling really lost on one area. I'm getting the formula kind of/sort of, but -- where does the 367 come from? Why wouldn't it be 366*366?
So sorry to sound dumb, but I honestly want to get this!!
@owlwings (43910)
• Cambridge, England
1 Jan 16
@mawhite Going by the formula alone (without understanding why it is how it is - we can deal with that later) then if n=366, n+1 must be 367.
It's worth noting that the sum of a series of integers must be an integer, so the top part of the formula (the bit which has to be divided by two) must be an even number. Conveniently, if you take any number (odd or even) and multiply it by the same number plus one, you always end up with an even number - even times odd = even.
2 people like this
@mawhite (242)
• United States
1 Jan 16
@owlwings This has been an interesting experience, far more than the fun Facebook meme it started out as.
It really threw me back years memory-wise, remembering algebra in high school, and how I felt like I was 'almost there' understanding it, but there was this fixation/block on not understanding "why", if that makes any sense.
Do you know, I honestly have been surfing math tutoring sites for the past hour since your comment!
It was like I could do the formula itself standing on my head, but there was this part of me that was so hung up on: "Why (n*(n+1))/2 and not just (n*n)/2"
When I saw it visualized on a math site about taking the triangle, doubling & flipping it to make a rectangle, and then seeing that when this is done, THAT'S where the extra column comes from, that it started to make sense for me.
In any event, THANK YOU, THANK YOU, THANK YOU for tuning a mundane Facebook share that I happened across into such an interesting start to the New Year!!
2 people like this
@softbabe44 (5816)
• Vancouver, Washington
1 Jan 16
That's a good way to get the best answer are you doing this at this time or not.
1 person likes this
@Marilynda1225 (83103)
• United States
1 Jan 16
Sounds like a pretty easy way to save up $667
1 person likes this
@fishtiger58 (29820)
• Momence, Illinois
1 Jan 16
Math is not my strong suit either. Seems you got some good answers here. And that's a good thing. I sure couldn't help you. Good luck with your saving.
1 person likes this
@Morleyhunt (21744)
• Canada
18 Jan 16
No pennies in canada
. I'll need to do it with another coin....one I have lots of we'll see how it goes.
. I'll need to do it with another coin....one I have lots of we'll see how it goes.